Real Analysis 2000/-
Syllabus –
Analysis: Elementary set theory, finite, countable and uncountable sets, Real number system as a complete ordered field, Archimedean property, supremum, infimum. Sequences and series, convergence, limsup, liminf. Bolzano Weierstrass theorem, Heine Borel theorem. Continuity, uniform continuity, differentiability, mean value theorem. Sequences and series of functions, uniform convergence. Riemann sums and Riemann integral, Improper Integrals. Monotonic functions, types of discontinuity, functions of bounded variation, Lebesgue measure, Lebesgue integral. Functions of several variables, directional derivative, partial derivative, derivative as a linear transformation, inverse and implicit function theorems. Metric spaces, compactness, connectedness. Normed linear Spaces. Spaces of continuous functions as examples.
Linear Algebra 1800/-
Syllabus –
Linear Algebra: Vector spaces, subspaces, linear dependence, basis, dimension, algebra of linear transformations. Algebra of matrices, rank and determinant of matrices, linear equations. Eigenvalues and eigenvectors, Cayley-Hamilton theorem. Matrix representation of linear transformations. Change of basis, canonical forms, diagonal forms, triangular forms, Jordan forms. Inner product spaces, orthonormal basis. Quadratic forms, reduction and classification of quadratic forms.
Complex Analysis 1500/-
Syllabus –
Algebra of complex numbers, the complex plane, polynomials, power series, transcendental functions such as exponential, trigonometric and hyperbolic functions. Analytic functions, Cauchy-Riemann equations. Contour integral, Cauchy’s theorem, Cauchy’s integral formula, Liouville’s theorem, Maximum modulus principle, Schwarz lemma, Open mapping theorem. Taylor series, Laurent series, calculus of residues. Conformal mappings, Mobius transformations.
Algebra 2000/-
Syllabus –
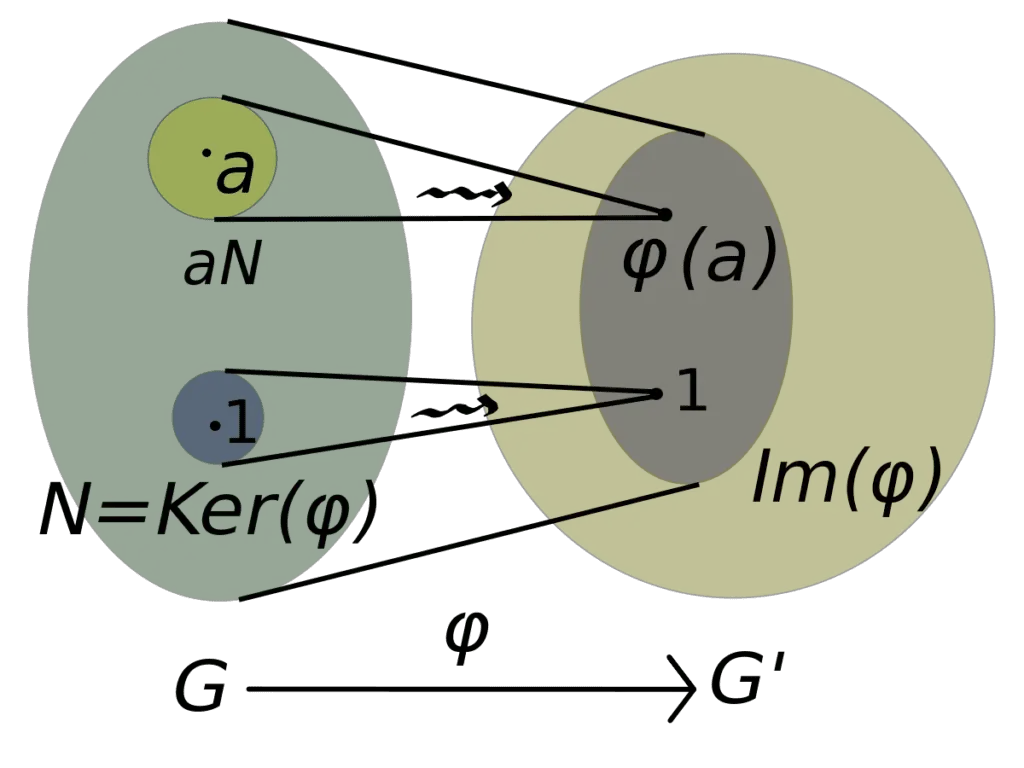
Algebra: Permutations, combinations, pigeon-hole principle, inclusion-exclusion principle, derangements. Fundamental theorem of arithmetic, divisibility in Z, congruences, Chinese Remainder Theorem, Euler’s Ø- function, primitive roots. Groups, subgroups, normal subgroups, quotient groups, homomorphisms, cyclic groups, permutation groups, Cayley’s theorem, class equations, Sylow theorems. Rings, ideals, prime and maximal ideals, quotient rings, unique factorization domain, principal ideal domain, Euclidean domain. Polynomial rings and irreducibility criteria. Fields, finite fields, field extensions, Galois Theory.
Topology 1000/-
Syllabus –
Analysis: Elementary set theory, finite, countable and uncountable sets, Real number system as a complete ordered field, Archimedean property, supremum, infimum. Sequences and series, convergence, limsup, liminf. Bolzano Weierstrass theorem, Heine Borel theorem. Continuity, uniform continuity, differentiability, mean value theorem. Sequences and series of functions, uniform convergence. Riemann sums and Riemann integral, Improper Integrals. Monotonic functions, types of discontinuity, functions of bounded variation, Lebesgue measure, Lebesgue integral. Functions of several variables, directional derivative, partial derivative, derivative as a linear transformation, inverse and implicit function theorems. Metric spaces, compactness, connectedness. Normed linear Spaces. Spaces of continuous functions as examples.
